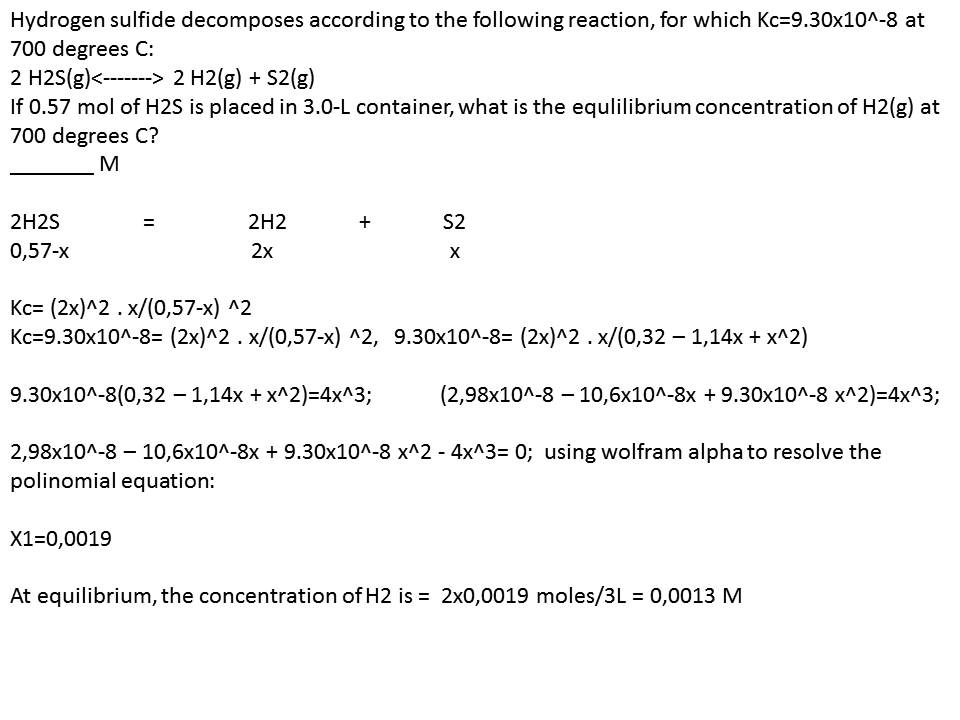
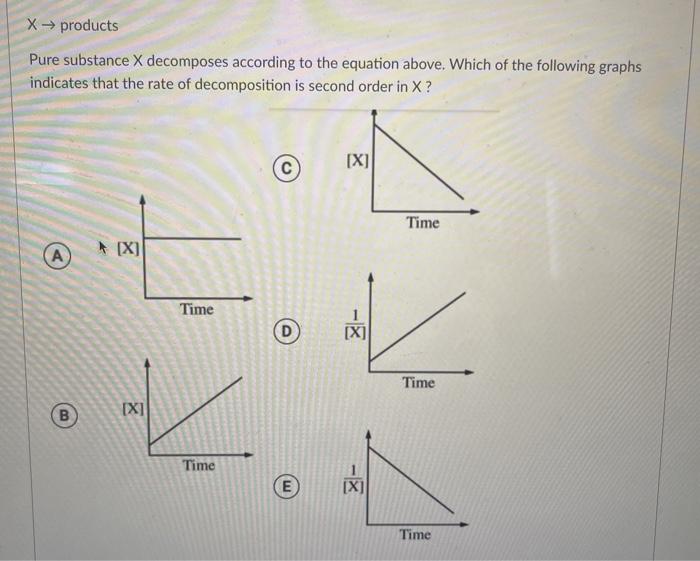
Pure substance X decomposes according to the equation above, embarking us on a scientific expedition to decipher the intricacies of this chemical transformation. This decomposition reaction holds significant implications, offering insights into the behavior of matter and its applications in various fields.
The decomposition equation provides a roadmap for the reaction, revealing the reactants, products, and their stoichiometric relationship. Delving deeper, we explore the reaction mechanism, uncovering the intricate steps and energy barriers involved. Thermodynamics unveils the energetics of the process, determining whether the reaction is spontaneous or not.
Decomposition Equation: Pure Substance X Decomposes According To The Equation Above
The decomposition of pure substance X is represented by the following equation:
X → 2Y + Z
In this equation, X is the reactant, Y and Z are the products, and the stoichiometry indicates that 2 moles of X decompose to produce 2 moles of Y and 1 mole of Z.
Reaction Mechanism, Pure substance x decomposes according to the equation above
A possible mechanism for the decomposition reaction is as follows:
- Step 1:X → X* (formation of an excited intermediate)
- Step 2:X* → Y + Z (decomposition of the excited intermediate)
The activation energy for the reaction is the energy required to form the excited intermediate, and the rate-determining step is the decomposition of the excited intermediate.
Thermodynamics of Decomposition
The enthalpy change (ΔH) for the decomposition reaction can be calculated using bond energies:
ΔH = (Bond energy of X-X)
(Bond energy of Y-Y + Bond energy of Z-Z)
If ΔH is positive, the reaction is endothermic, and if ΔH is negative, the reaction is exothermic. The spontaneity of the reaction is determined by the Gibbs free energy change (ΔG), which is related to ΔH and the entropy change (ΔS) by the equation:
ΔG = ΔH
TΔS
where T is the temperature in Kelvin.
Kinetics of Decomposition
The rate law for the decomposition reaction is:
Rate = k[X]^2
where k is the rate constant. The reaction is second order with respect to the reactant X. The effects of temperature and concentration on the reaction rate can be explained using the Arrhenius equation and the rate law, respectively.
Applications of Decomposition
The decomposition reaction of pure substance X has several practical applications:
- Industrial processes:The decomposition of X is used in the production of chemicals, such as hydrogen and oxygen.
- Scientific techniques:The decomposition of X is used in analytical chemistry to determine the concentration of X in a sample.
- Environmental science:The decomposition of X is used to remove pollutants from the environment.
FAQ
What is the significance of the decomposition equation?
The decomposition equation provides a quantitative description of the reaction, allowing us to determine the stoichiometry, predict product yields, and understand the chemical changes occurring.
How does the reaction mechanism influence the decomposition process?
The reaction mechanism reveals the detailed steps and energy barriers involved in the decomposition, providing insights into the reaction pathway and the factors affecting its rate.
What practical applications are derived from the decomposition of pure substance X?
The decomposition reaction has applications in various fields, including the production of hydrogen fuel, thermal analysis techniques, and the synthesis of new materials.