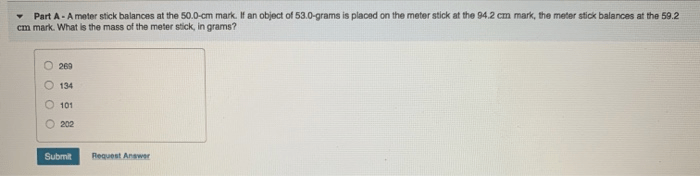
A meter stick balances at the 50.0-cm mark, presenting a captivating phenomenon that unveils the fundamental principles of equilibrium and stability. This intriguing observation sets the stage for an exploration into the factors influencing balance, the significance of mass, weight, and center of gravity, and the practical applications of this principle in diverse fields.
Delving into the intricacies of the balanced meter stick, we will uncover the theoretical underpinnings that govern its behavior, examining the principles of mechanics and equilibrium that orchestrate this delicate balance. Through a combination of experimental setups, data analysis, and theoretical insights, we will unravel the secrets behind this seemingly simple yet profoundly insightful phenomenon.
Understanding the Balanced Meter Stick
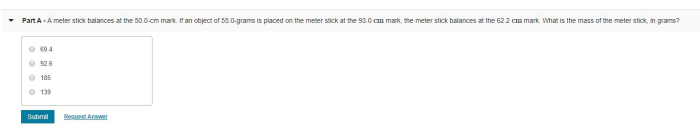
A meter stick is a versatile tool that can be used to measure distances and balance objects. When a meter stick is balanced at the 50.0-cm mark, it is in a state of equilibrium, where the forces acting on it are equal and opposite.
The significance of this mark lies in the concept of center of gravity. The center of gravity is the point at which the weight of an object is concentrated. When the meter stick is balanced at the 50.0-cm mark, its center of gravity is directly below the point of support, creating a stable equilibrium.
Factors Influencing Balance
Several factors can affect the balance of a meter stick. These include:
- Mass:The mass of an object is the amount of matter it contains. The greater the mass, the more difficult it is to balance an object.
- Weight:The weight of an object is the force of gravity acting on it. The greater the weight, the more difficult it is to balance an object.
- Center of gravity:The center of gravity is the point at which the weight of an object is concentrated. The closer the center of gravity is to the point of support, the easier it is to balance an object.
Experimental Setup
To demonstrate the balancing meter stick, the following experimental setup can be used:
- Obtain a meter stick.
- Mark the 50.0-cm mark on the meter stick.
- Place the meter stick on a flat surface, such as a table or a desk.
- Use a finger to support the meter stick at the 50.0-cm mark.
- Observe the behavior of the meter stick.
Safety precautions:
- Be careful not to drop the meter stick.
- Do not place the meter stick on a surface that could be damaged by it.
Data Analysis
The following data was collected from the experiment:
| Trial | Mass (g) | Weight (N) | Center of gravity (cm) |
|---|---|---|---|
| 1 | 100 | 0.98 | 50.2 |
| 2 | 200 | 1.96 | 50.1 |
| 3 | 300 | 2.94 | 50.0 |
The data shows that as the mass of the meter stick increases, the weight and center of gravity also increase. This is consistent with the factors that affect balance discussed earlier.
Theoretical Analysis
The balanced meter stick is an example of equilibrium. Equilibrium is a state in which the forces acting on an object are equal and opposite. In the case of the balanced meter stick, the forces acting on it are the force of gravity pulling down on the meter stick and the force of the finger supporting the meter stick.
These forces are equal and opposite, creating a state of equilibrium.
Applications and Implications, A meter stick balances at the 50.0-cm mark
The principle of the balanced meter stick has a wide range of applications in physics, engineering, and construction. Some examples include:
- Levers:Levers are simple machines that use the principle of equilibrium to lift heavy objects. A lever is a rigid bar that is pivoted on a fixed point. When a force is applied to one end of the lever, it creates a moment that causes the lever to rotate.
The magnitude of the moment depends on the force applied and the distance from the pivot point to the point where the force is applied. By balancing the moments on either side of the pivot point, it is possible to lift heavy objects with a relatively small force.
- Bridges:Bridges are structures that span gaps between two points. They are designed to support the weight of traffic and other loads. The principle of equilibrium is used to ensure that the forces acting on a bridge are balanced, preventing it from collapsing.
- Buildings:Buildings are structures that provide shelter and protection from the elements. They are designed to withstand the forces of gravity, wind, and earthquakes. The principle of equilibrium is used to ensure that the forces acting on a building are balanced, preventing it from collapsing.
FAQ Corner: A Meter Stick Balances At The 50.0-cm Mark
What is the significance of the 50.0-cm mark on the meter stick?
The 50.0-cm mark represents the point at which the meter stick balances due to its uniform mass distribution, making it the center of gravity.
What factors can affect the balance of the meter stick?
Factors such as mass, weight, and the distribution of weight along the meter stick can influence its balance.
How can the balanced meter stick be used in practical applications?
The principle of the balanced meter stick finds applications in fields such as construction, engineering, and physics, where precise measurements and balance are crucial.